Any unit Frechet max-stable process can be arbitrarly well approximated by a max-linear model by taking sufficiently large
Max-stable processes
Conditional max-stable simulations
Latent Variable
Copula approaches
Miscellaneous
References
Reference Manual
What is a max-linear model?
A unit Frechet max-linear process is defined by
 =
\max_{j=1, \ldots, p} f_j(x) Z_j, \qquad x \in \mathbb{R}^d,)
where 
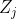are
independent unit Frechet random variables
and 
are
non-negative deterministic functions such that
 = 1, \qquad x \in \mathbb{R}^d.)
Any unit Frechet max-stable process can be arbitrarly well approximated by a max-linear model by taking sufficiently large
and
suitable
functions .
Any unit Frechet max-stable process can be arbitrarly well approximated by a max-linear model by taking sufficiently large
Function "condrmaxstab": Performs conditional simulations for max-stable processes
Since the
functions
are deterministics, Wang and Stoev [2011] proposed an efficient
algorithm to generate conditional simulations from a max-linear
model and thus approximate conditional simulations from a
max-stable process.
However this approach has some drawbacks since it is not clear how to find appropriate functions. Therefore
the only available model is currently the (discretized) Smith
model for which
 =
c(p) \varphi(x - u_j ; \Sigma), \qquad x \in \mathbb{R}^d,)
where )
is the zero mean (multivariate) normal density with covariance
matrix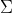 
, 
are appropriately chosen points
in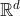 
and )
is a
constant ensuring unit Frechet margins.
However this approach has some drawbacks since it is not clear how to find appropriate functions